Waldhausen category
In mathematics, a Waldhausen category is a category C equipped with some additional data, which makes it possible to construct the K-theory spectrum of C using a so-called S-construction. It's named after Friedhelm Waldhausen, who introduced this notion (under the term category with cofibrations and weak equivalences) to extend the methods of algebraic K-theory to categories not necessarily of algebraic origin, for example the category of topological spaces.
Definition
Let C be a category, co(C) and we(C) two classes of morphisms in C, called cofibrations and weak equivalences respectively. The triple (C, co(C), we(C)) is called a Waldhausen category if it satisfies the following axioms, motivated by the similar properties for the notions of cofibrations and weak homotopy equivalences of topological spaces:
- C has a zero object, denoted by 0;
- isomorphisms are included in both co(C) and we(C);
- co(C) and we(C) are closed under composition;
- for each object A ∈ C the unique map 0 → A is a cofibration, i.e. is an element of co(C);
- co(C) and we(C) are compatible with pushouts in a certain sense.
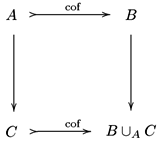
For example, if [math]\displaystyle{ \scriptstyle A\, \rightarrowtail\, B }[/math] is a cofibration and [math]\displaystyle{ \scriptstyle A\,\to\, C }[/math] is any map, then there must exist a pushout [math]\displaystyle{ \scriptstyle B\, \cup_A\, C }[/math], and the natural map [math]\displaystyle{ \scriptstyle C\, \rightarrowtail\, B\,\cup_A\, C }[/math] should be cofibration:
Relations with other notions
In algebraic K-theory and homotopy theory there are several notions of categories equipped with some specified classes of morphisms. If C has a structure of an exact category, then by defining we(C) to be isomorphisms, co(C) to be admissible monomorphisms, one obtains a structure of a Waldhausen category on C. Both kinds of structure may be used to define K-theory of C, using the Q-construction for an exact structure and S-construction for a Waldhausen structure. An important fact is that the resulting K-theory spaces are homotopy equivalent.
If C is a model category with a zero object, then the full subcategory of cofibrant objects in C may be given a Waldhausen structure.
S-construction
The Waldhausen S-construction produces from a Waldhausen category C a sequence of Kan complexes [math]\displaystyle{ S_n(C) }[/math], which forms a spectrum. Let [math]\displaystyle{ K(C) }[/math] denote the loop space of the geometric realization [math]\displaystyle{ |S_*(C)| }[/math] of [math]\displaystyle{ S_*(C) }[/math]. Then the group
- [math]\displaystyle{ \pi_n K(C) = \pi_{n+1} |S_*(C)| }[/math]
is the n-th K-group of C. Thus, it gives a way to define higher K-groups. Another approach for higher K-theory is Quillen's Q-construction.
The construction is due to Friedhelm Waldhausen.
biWaldhausen categories
A category C is equipped with bifibrations if it has cofibrations and its opposite category COP has so also. In that case, we denote the fibrations of COP by quot(C). In that case, C is a biWaldhausen category if C has bifibrations and weak equivalences such that both (C, co(C), we) and (COP, quot(C), weOP) are Waldhausen categories.
Waldhausen and biWaldhausen categories are linked with algebraic K-theory. There, many interesting categories are complicial biWaldhausen categories. For example: The category [math]\displaystyle{ \scriptstyle C^b(\mathcal{A}) }[/math] of bounded chain complexes on an exact category [math]\displaystyle{ \scriptstyle \mathcal{A} }[/math]. The category [math]\displaystyle{ \scriptstyle S_n \mathcal{C} }[/math] of functors [math]\displaystyle{ \scriptstyle \operatorname{Ar}(\Delta ^n)\, \to\, \mathcal{C} }[/math] when [math]\displaystyle{ \scriptstyle\mathcal{C} }[/math] is so. And given a diagram [math]\displaystyle{ \scriptstyle I }[/math], then [math]\displaystyle{ \scriptstyle \mathcal{C}^I }[/math] is a nice complicial biWaldhausen category when [math]\displaystyle{ \scriptstyle \mathcal{C} }[/math] is.
References
- Waldhausen, Friedhelm (1985), "Algebraic K-theory of spaces", Algebraic and geometric topology (New Brunswick, N.J., 1983, Lecture Notes in Mathematics, 1126, Berlin: Springer, pp. 318–419, doi:10.1007/BFb0074449, ISBN 978-3-540-15235-4, http://www.maths.ed.ac.uk/~aar/surgery/rutgers/wald.pdf
- C. Weibel, The K-book, an introduction to algebraic K-theory — http://www.math.rutgers.edu/~weibel/Kbook.html
- G. Garkusha, Systems of Diagram Categories and K-theory — https://arxiv.org/abs/math/0401062
- Sagave, S. (2004). "On the algebraic K-theory of model categories". Journal of Pure and Applied Algebra 190 (1–3): 329–340. doi:10.1016/j.jpaa.2003.11.002.
- Lurie, Jacob, Higher K-Theory of ∞-Categories (Lecture 16), http://www.math.harvard.edu/~lurie/281notes/Lecture16-Higher.pdf
See also
- Complete Segal space
External links
 |